finding the equation of the tangent to e^2x at x=1

[pvtponcho]

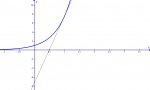
I have tried to work out this problem, but it honestly don't know how to tackle this at all.
I think i have to use 1 in the equation to get the y intercept, but i'm not exactly sure. After that I am kind of clueless. Any help would be much appreciated.
Thanks!!

 

[tkhunny]

There is no need for multiple posting.

Do you mean \(\displaystyle y = e^{2x}\) or \(\displaystyle y = e^{2}x\)?

What is the first derivative of the correct function? Show your work.

 

[pvtponcho]

the first derivative would be 2e^(2x) because of the derivative of e is itself times the derivative of its exponent. e^(2x) times 2.

 

[tkhunny]

I thought you would figure out the Moderated Post thing. That should stop after a few successful psots.

Okay, you have the derivative. What will you be doing with it? When evaluated for a particular value of 'x', as what do we interpret this value?

 

[galactus]

Sub x=1 into \(\displaystyle y=e^{2x}\) to find the y value at x=1. You now have x and y.

After finding the derivative (slope) at x=1, you now have x,y, and m.

Sub them into y=mx+b, solve for b, and you're done.

Also, graph it as to give an idea if you're in the ballpark.