This problem seems very hard to me. I'd like to figure it out on my own but a nudge in the right direction would be great.
Find a polynomial function,
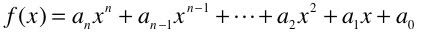
thats has only the specified extrema:
Relative minima: (0,0), (4,0)
Relative maximum: (2,4)
(A) Determine the minimum degree of the function and give the criteria you used in determining the degree.
I believe that the degree must be at least 4, since there are three critical numbers.
(B) Using the fact that the coordinates of the extrema are solution points of the function, and that the x-coordinates are critical numbers, determine a system if linear equations whose solution yields the coefficients of the required function.
So i plugged it in and got this:
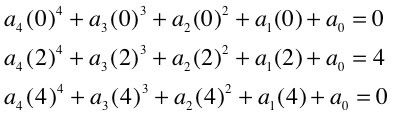
I feel as if im close but i don't know, and i really dont know what to do with these equations. The rest of the problem states:
(C)Use a graphing utility to solve the system of equations and determine the function. (D) Use a graphing utility to confirm your results graphically.
Thanks for any help you can give me. If it's useful to know my calculator is a TI-89.
Find a polynomial function,
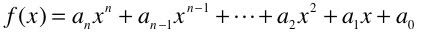
thats has only the specified extrema:
Relative minima: (0,0), (4,0)
Relative maximum: (2,4)
(A) Determine the minimum degree of the function and give the criteria you used in determining the degree.
I believe that the degree must be at least 4, since there are three critical numbers.
(B) Using the fact that the coordinates of the extrema are solution points of the function, and that the x-coordinates are critical numbers, determine a system if linear equations whose solution yields the coefficients of the required function.
So i plugged it in and got this:
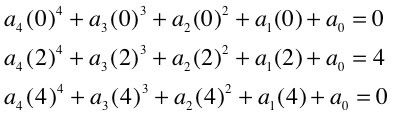
I feel as if im close but i don't know, and i really dont know what to do with these equations. The rest of the problem states:
(C)Use a graphing utility to solve the system of equations and determine the function. (D) Use a graphing utility to confirm your results graphically.
Thanks for any help you can give me. If it's useful to know my calculator is a TI-89.
