Finding Efficient Shape to Maximize Volume

M

mathnerd

New member
Joined
Apr 9, 2008
Messages
11
Hey guys, I need help in solving this question. If you could please start me off with some hints, I will try solving it. Thanks.

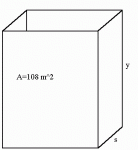
An open-topped storage box is to have a square base and vertical sides. If 108 m^2 of sheet metal is available for its construction, find the most efficient shape to maximize it's volume.

Please help out on this hard calculus question. Thank you.
 
mathnerd said:
An open-topped storage box is to have a square base and vertical sides. If 108 m^2 of sheet metal is available for its construction, find the most efficient shape to maximize it's volume.
Click to expand...
What have you tried? How far did you get? Where are you stuck? You draw the box, picked a variable for the side-lengths of the base, labelled the picture, wrote down the formulas for volume and surface area, and... then what?

Please be complete. Thank you! :D

Eliz.
 
Sorry. Here is what I did:

[attachment=0:2dqkb8xf]image92.GIF[/attachment:2dqkb8xf]

And then, the side length of the square base is with the variable 's'. I have labeled the picture.

The formula for the area of a cube is s^2
The formula for area of rectangle is a*b

But, I don't understand the fact that the vertical sides are rectangles or cubes too.

The volume of a cube is s^3
The volume of a rectangular prism is V =lwh

Surface area of a cube is 6a^2
Surface area of a rectangular prism is 2(wh+lw+lh).

I think it would be helpful for me, maybe if you could explain the question to me. The question is too vague. Also, I think 108 m^2 is the surface area, but I don't know how to apply it to get maximum volume.

Please help. Thanks.
 

Attachments

  • image92.GIF
    image92.GIF
    3 KB · Views: 543
Thanks Dr. Flim-Flam.

Here is what I did.

s^2 + 4sy = 108
y = (108-s^2) / 4s

plug this "y" into volume formula

V = s^2 (y)
= s^2 ((108-s^2) / 4s)
= ( 108s^2 - s^4 ) / 4s
108s^2 = s^4
108 = s^2
s = 108^0.5

Is this right? Should I continue working this out, meaning now that I have found s, I can find y, Then plug the y and x into volume formula to get the maximized volume?

But, I don't get why the question says "the most efficient shape". Am I on the right track. Please help. Thanks.
 
Thanks very much for your help. After differentiating V(s), and putting V(s)` = 0, the value of s is 6 m

Then, I found the value of y to be 3 m.

The volume comes out to be 108 m^3 (somehow it's the same as the area)

So, is this the answer? Because the question says 'the most efficient' shape. Could I get some more help on that? Thanks.
 
lol. Thanks very much. You are a great helper. Doing this question was fun. Thanks.
 
You must log in or register to reply here.
Top