Finding Area Bounded by Graphs

[alyssa23851]

I was hoping I could get some help on this. I have it worked down until nearly the end, but I can't figure out what to do or if I'm even doing this right because I haven't used e's since the begining of this year.

The instructions are as follows:

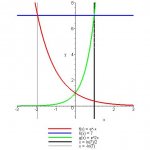
Find the area bounded by the graph of f(x) = e^-x , g(x) = e^2x , and h(x) =7.

For my bounds, I have -ln7 to 0 for h(x) and f(x), and 0 to (ln7)/2 for h(x) and g(x).

For the intergral f(x) and h(x), I have 7-3^-x.

I took the derivative:
7x+e^-x from -ln7 to 0


For the intergral of g(x) and h(x), I have 7-e^2x.

I took the derivative:
7x-1/2e^2x from 0 to ln7/2

and combined them, plugging in my bounds:

[{7(0)+e^-0}-{7(-ln7)+e^ln7}]+[{7(ln7)/2-1/2e^[(ln7)/2]}-{7(0)+e^-0]

Of course, I went by the right order of operations.
From here, I don't know what to do. Because I keep checking my work with another website, and I cannot understand how they got the answer they did. I know it looks confusing, but I would greatly appreciate some help. Thankyou so much!

 

[BigGlenntheHeavy]

\(\displaystyle A \ = \ \int_{-ln7}^{0}[7-e^{-x}]dx \ + \ \int_{0}^{ln7/2}[7-e^{2x}]dx \ = \ \frac{21ln7}{2}-9 \ sq. \ units, \ (x-axis).\)

\(\displaystyle A \ = \ \frac{3}{2}\int_{1}^{7}[lny] dy \ = \ \frac{21ln7}{2}-9 \ sq. \ units, \ (y-axis).\)

\(\displaystyle Y-axis \ is \ easier, \ only \ one \ integral.\)

\(\displaystyle See \ graph.\)

[attachment=0:zzp8ivpf]zde.jpg[/attachment:zzp8ivpf]