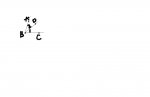
Find m<ABC if line BD bisects <ABC,m<=5x-11 and m<2=3x+5

[Jeff24]

Find m<ABC if line BD bisects <ABC, m<1=5x-11, and m<2=3x+5[attachment=0:1hka45l1]geometry 5.JPG[/attachment:1hka45l1]

I don't understand how to set it up , or even start the problem, what would I do how do I know my answers right?

 

[mmm4444bot]

(I'm wondering whether or not MathIQ is the same person as Jeff24.)

The word "bisect" is a verb. It means to cut something into two EQUAL pieces.

In other words, if an angle is bisected, then it is divided into two smaller angles which are both equal.

If I bisect an angle of 90 degrees, then the two resulting angles each measure 45 degrees.

If I bisect an angle of 100 degrees, then the two resulting angles each measure 50 degrees.

If I bisect angle ABC, then the two resulting angles each measure the same.

What does this tell you about the relationship between the following two expressions?

5x - 11

3x + 5

Use the relationship to write an equation.

Solve this equation for x.

Substitute this value for x into either expression (it does not matter which because the value of both expressions is the same).

Once you know the measure of either angle 1 or 2, then double it to find the measure of angle ABC.

 

[MathIQ]

Re: Find m<ABC

So I would set it up as 5x-11=3x+5
But when would i use the degree's of this angle in this equation would it be 5x-11=3x+5=____ degrees and how would i know how many degress it is becasue it's not right or abtuse , it's acute so would i use 90 degrees?

 

[mmm4444bot]

So I would set it up as 5x-11=3x+5 Yes!

But when would i use the degree's of this angle in this equation ... ? After you solve for x.

Solve the equation for x.

Take that value, and substitute it for x in the expression 3x + 5. The number you get is the measure of the two angles that make up angle ABC.

So, double it.

The result is the measure of angle ABC.

Here's a similar example.

Some angle called DEF is bisected into angles 1 and 2.

The measure of angle 1 is given by the expression 7x - 12.

The measure of angle 2 is given by the expression 4x - 5.

What is the measure of angle DEF?

SOLUTION:

Since angle DEF has been "bisected", the measures of angles 1 and 2 are equal. So, we set their expressions equal to each other.

7x - 12 = 4x - 5

Solve for x.

x = 7/3

Substitute the value 7/3 for x in either the expression for the measure of angle 1 OR the expression for the measure of angle 2. (It does not matter which expression you use because they both represent the same measure.)

I choose to use the expression for angle 1.

7x - 12

I substitute 7/3 for x.

7(7/3) - 12

Now, I do the arithmetic to find the measure of angle 1.

49/3 - 12

49/3 - 36/3

(49 - 36)/3

13/3

The measure of angle 1 is 13/3 degrees.

Since angle 1 is exactly ONE HALF of angle DEF, we can get the measure of angle DEF by doubling 13/3.

2(13/3) = 26/3

The measure of angle DEF is 26/3 degrees.

Do you see how this example corresponds to your exercise?

If you do, then follow the same strategy.

If you do not, then explain the reason why, and I'll go from there.

 

[MathIQ]

Re: Find m<ABC

5x-11=3x+5
-3x+11-3x+11
___________
2x=16
__ __
2 2

x=8

Then do I times 8 by 2 because i'm trying to find the value of m<ABC
So i get 16?

 

[mmm4444bot]

x=8 <<< This is correct.

So i get 16? <<< This does not look right.

If the measure of angle ABC is 16 degrees, then the measures of angles 1 and 2 are both 8 degrees, right?

The measure of angle 1 is 5x - 11. But, this expression does not equal 8 when x is 8.

The measure of angle 2 is 3x + 5. But, this expression does not equal 8 when x is 8.

Therefore, the measure of angle ABC is not 16 degrees.

Why don't you try following the instructions that I gave you, instead?

 

[MathIQ]

Re: Find m<ABC

I just don't understand, we found out what <1 and <2 is.
So <1 and <2 make up <ABC
Can you give me anymore hints I looked at your example over again and again and it still isn't coming to me!

 

[MathIQ]

So I put it all together and got
5x-11=3x+5
-3x+11-3x+5
___________
2x=16

x=8

Is ther anything I have to do after this?

 

[MathIQ]

[attachment=0:2q8kjixr]geometry 22.JPG[/attachment:2q8kjixr]

Is x the answer,or do I have to times something by x to get the answer?

 

[Denis]

You're asked for the measure of angle ABC, which is made up of 5x-11 and 3x+5,
so angle ABC = 5x - 11 + 3x + 5 = 8x - 6.
You already know that x = 8, so what's your problem?
Do us a favor: start THINKING a bit; you'll be doing yourself a favor too :idea:

 

[mmm4444bot]

This discussion looks very familiar, to me. Jeff might be conducting this discussion in two different locations.

It looks like a duplicate thread, but I'm not sure because I've lost track.

 

[mmm4444bot]

… start THINKING a bit …

Denis, there might be some type of organic neural deficit at work here. I think the original poster probably needs face-to-face tutoring by somebody qualified to get to the root of the issue.

 

[stapel]

Duplicate threads merged.