find lines simultaneously tangent to 6-10x^2 and 8-(x-2)^2

[skatru]

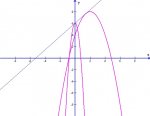
The graphs of the quadratic functions

f(x) = 6-10x^2

and

g(x)=8-(x-2)^2

Find the lines simultaneously tangent to both graphs.

I know I need to find some version of y=m(x-a)+b. I know how to find the m but I don't know how to the points where the graphs intersect.

Thank you for any help provided.

 

[galactus]

Re: Stuck

Let $\displaystyle A(a, 6-10a^{2})$ be a point on $\displaystyle y=6-10x^{2}$

It has derivative(slope) of $\displaystyle y'=-20x$

The slope at $\displaystyle x=a$ is $\displaystyle -20a$


Let $\displaystyle B(b, 8-(b-2)^{2})$ be a point on $\displaystyle y=8-(x-2)^{2}$

It has derivative of $\displaystyle y'=4-2x$

The slope at $\displaystyle x=b$ is $\displaystyle 4-2b$

The slopes have to be equal, so $\displaystyle -20a=4-2b$----------->$\displaystyle b=10a+2$.........[1]

The slope of AB is $\displaystyle \frac{(6-10a^{2})-(8-(b-2)^{2})}{a-b}=\frac{-10a^{2}+b^{2}-4b+2}{a-b}$

This has to equal the slope at A:

$\displaystyle \frac{-10a^{2}+b^{2}-4b+2}{a-b}=-20a$

$\displaystyle \frac{-9b^{2}}{a-b}-\frac{4b}{a-b}+\frac{2}{a-b}+10a-10b=0$

Sub in [1] to get it into one variable and get:

$\displaystyle \frac{-22}{9(9a+2)}+10a+\frac{20}{9}=0$

Now, solve for a and b will follow. Can you finish?. Make sure my algebra is good.

Perhaps we could've taken a less messy course, but hopefully you get the idea.

Here's a graph of one of the lines:

 

[skatru]

Thanks