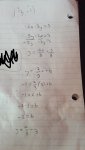equation of line parallel to 2x - 3y = 5 containing point (3, -1)

[mech649]

Find the equation of the line that contains the point (3,-1) and is parallel to the line 2x-3y = 5

 

[stapel]

Find the equation of the line that contains the point (3,-1) and is parallel to the line 2x-3y = 5

What is the slope (here) of the original line, 2x - 3y = 5?

Given this slope, and using the given point, what line equation (here) did you get?

Please be complete. Thank you!

 

[mech649]

Here is how the book phrased it. Thank you!!!

 

[stapel]

Here is how the book phrased it. Thank you!!!

Yes. Now, what are your answers to the questions I'd asked of you?

Please be complete. Thank you!

 

[Dr Fua]

Find the equation of the line that contains the point (3,-1) and is parallel to the line 2x-3y = 5

the slope of a linear equation is equal to the slope of a parallel linear equation.

 

[mech649]

here is my work. my answer seems to be different from the answers on the back of the book.

 

[stapel]

here is my work.

$\displaystyle 2x\, -\, 3y\, =\, 5$

$\displaystyle \dfrac{3y}{+3y}\, -\, \dfrac{2x}{+3y}\, +\, \dfrac{5}{+3}$

Where on earth did the second line come from? :shock:

Let's start smaller. If they'd given you the following:

. . . . .$\displaystyle 2\, -\, 3y\, =\, 5$

...how would you have solved this for "y="?

Please show all of your steps. Thank you!

 

[pka]

Find the equation of the line that contains the point (3,-1) and is parallel to the line 2x-3y = 5

I for one cannot read anything in the image that you posted in #3. I wish people could or would type problems out.

This is always the case however:
The line parallel to $\displaystyle ax+by+c=0$ that contains $\displaystyle (p,q)$ is
$\displaystyle \large ax+by-(ap+bq)=0$.