Cylindrical coordinates

[njmiano]

Hi,
I am having problems with a homework question,

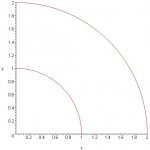
I am asked to sketch the solid by the given inequalities.

0 <= theta <= pi/2 and r <= z <= 2

How do I find r?

If possible please guide me to the answer instead of giving it to me. I need to know this stuff.

Thanks in advance for any help.

 

[daon]

In cylindrical coordinates, given \(\displaystyle P(x,y)\), \(\displaystyle r_P:=d(\big{O}, (x,y)) = \sqrt{x^2+y^2}\)

When defined, these are also available: \(\displaystyle r = \frac{x}{cos \theta} = \frac{y}{sin \theta}\), where \(\displaystyle \theta=\tan^{-1}\frac{y}{x}\)

Your solid is in Octant 1, \(\displaystyle x,y,z \ge 0\).

Instead of using \(\displaystyle r \le z \le 2\), use \(\displaystyle x^2+y^2 \le z^2 \le 4\)

It would be a good idea to know the graph of the equation \(\displaystyle z=r\) (and several more) by heart.

 

[BigGlenntheHeavy]

[attachment=0:16bz91bo]xyz.jpg[/attachment:16bz91bo]

The r value is from 0 to 1 on the x axis and the z value is next.

Note: If you shaded in the area from x, y =1 to 2, that is your answer.