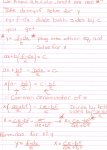ryan_kalle
New member
- Joined
- Jul 17, 2010
- Messages
- 17
Assignment 4
Find a formula to solve the system:
ax + by = c
dx + ey = f
where a,b,c,d,e and f are real numbers
Im a little confused on this one.
Our assignments are usually an application of previous material on our last test...sometimes.
We dealt with classifying and solving systems of equations using the addition/subraction and substitution method and finding unknowns when the product or sum is known. I'm not sure on how to solve this system with the current data.