Calculus two derivative questions.

[curlybit89]

Hello all!

I'm taking Calculus via an online course and am highly skeptical of all problems presented having personally found and confirmed 3 or 4 faulty problems (Eg a problem uses tan(3pi/6) in the denominator and the next step shows the denominator = 1).

I am taking care to record the problems that I absolutely could not understand and getting a second opinion of these problems.

Here is one of those problems now:

compute d/dx: (tan(x))/(1+x^2tan(x))

Two things come to mind, product rule and quotient rule. I am not certain about an order of operations regarding these rules go but I do see two instances of "x" in the denominator which indicates use of the product rule. Either way, my answers were wrong.

1) Quotient rule

((Sec(x)^2)+(x^2)(sec(x)^2)(tan(x) - (2x)(sec(x)^2)(tan)) / ((1+(x^2)(tan(x)))^2)

2) Product rule

((sec(x)^2))?? / ((2x)(tan(x)) + (x^2)(sec(x)^2))

----

QUESTION 2:

What is the rate of change of x with respect to Theta

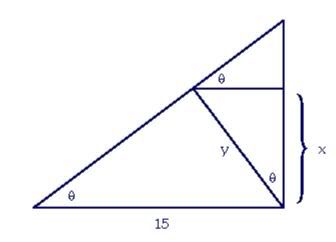
Right, I see three theta. I assume somehow one is suposed to work from theta (hyp = y) to theta (adj = 15). But I have no clue on how this is done, and if done, how one would determine the length of x to remove for the end triangle.

We cannout use theta where hyp = y because as theta changes x will remain the same and will not change for a rate of change of 0.

Any guidance provided here would be immensely appreciated.

 

[daon]

if you multiply the first through by cotx/cotx you'll get \(\displaystyle (cot(x)+x^2)^{-1}\). Then you may use the chain rule.

the fact that theta is displayed 3 times SHOULD mean all the theta are the same

oh, and use the following facts to find dtheta/dx:

ycos(theta)=x => cos(theta)=x/y (from the bottom right triangle).
15cos(pi/2-theta) = y => sin(theta)=y/15 (from the big triangle)

Then sin(theta)cos(theta) = 1/2sin(2theta) = x/15 => sin2theta = 2x/15

 

[BigGlenntheHeavy]

2nd problem:

\(\displaystyle sin(\theta) \ = \ \frac{y}{15}, \ y \ = \ 15sin(\theta)\)

\(\displaystyle cos(\theta) \ = \ \frac{x}{y}, \ = \ \frac{x}{15sin(\theta)}, \ hence \ x \ = \ 15sin(\theta)cos(\theta)\)

\(\displaystyle Ergo, \ \frac{dx}{d\theta} \ = \ 15[cos^{2}(\theta)-sin^{2}(\theta)]\)

\(\displaystyle Note: \ If \ we \ set \ the \ slope \ = \ to \ zero, \ then \ \theta \ = \ \frac{\pi}{4},\)

\(\displaystyle hence, \ x \ = \ its \ max \ = \ \frac{15}{2}.\)

\(\displaystyle Addendum: \ 15[cos^{2}(\theta)-sin^{2}(\theta)] \ = \ 0, \ (setting \ slope \ = \ to \ zero).\)

\(\displaystyle cos^{2}(\theta) \ = \ sin^{2}(\theta), \ cos(\theta) \ = \ sin(\theta), \ 0 \ < \ \theta \ < \ \frac{\pi}{2},\)

\(\displaystyle \frac{cos(\theta)}{cos(\theta)} \ = \ \frac{sin(\theta)}{cos(\theta)}, \ Ergo \ tan(\theta) \ =1, \ \theta \ = \ \frac{\pi}{4}.\)

\(\displaystyle Therefore \ x(\theta) \ = \ 15sin(\theta)cos(\theta), \ \implies \ x\bigg(\frac{\pi}{4}\bigg) \ = \ \frac{15}{2}.\)

 

[curlybit89]

2nd problem:

\(\displaystyle Note: \ If \ we \ set \ the \ slope \ = \ to \ zero, \ then \ \theta \ = \ \frac{\pi}{4},\)

\(\displaystyle hence, \ x \ = \ its \ max \ = \ \frac{15}{2}.\)

Hello Glenn.

I follow you completely up until this point, can you provide a little more context on setting the slope = 0 and why we would? As well as the rest of your answer. I'm a little muddled.

 

[BigGlenntheHeavy]

Setting the slope = to zero was just an afterthought, it really isn't necessary.

I just did it to show the max that x can achieve.

Note: See my addendum above.