akoaysigod
Junior Member
- Joined
- Oct 5, 2009
- Messages
- 65
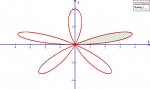
Find the area of the region enclosed by one loop of r = 4sin(5t)
which is...
t - 1/10(sin10t) which needs to be integrated from ? to ?
From the picture it looks like I should be able to integrate it from 0 to pi/6. I also tried pi/3 to 2pi/3 and going from 0 to 2pi and then multiplying by 1/5. None of them yield the correct response; I am pretty sure that I integrated it correctly.
which is...
t - 1/10(sin10t) which needs to be integrated from ? to ?
From the picture it looks like I should be able to integrate it from 0 to pi/6. I also tried pi/3 to 2pi/3 and going from 0 to 2pi and then multiplying by 1/5. None of them yield the correct response; I am pretty sure that I integrated it correctly.