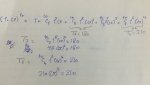Binomial expansion (1+cx)^10 , T_3=180, T_5=210 find c and x

[M4STR0k]

The point im stuck in :

 

[Harry_the_cat]

The point im stuck in :

I don't think this question makes any sense.

The coefficient in Term 3 will be 45c^2 = 180
The coefficient in Term 5 will be 210c^4 = 210
These are inconsistent!

And what does it mean by "find x"?

 

[pka]

I don't think this question makes any sense.
And what does it mean by "find x"?

Amen! I find that because there is little to no standard way to 'number' the terms of expansion, this question depends upon a particular set of notes. We do not have those notes.

Here is an example: Find the fourth term in the expansion of \(\displaystyle (x^2-x^{-1})^{10}\). Well what is the fourth term?

Had it been: Find the middle term in the expansion of \(\displaystyle (x^2-x^{-1})^{10}\) then that is definite.

 

[M4STR0k]

I don't think this question makes any sense.

The coefficient in Term 3 will be 45c^2 = 180
The coefficient in Term 5 will be 210c^4 = 210
These are inconsistent!

And what does it mean by "find x"?

Ye T5= 210 not coeff t5 =210