AS Student Question

[footytz]

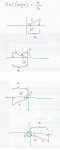
Hi, I am an AS Student. I wanted to ask you how do we calculate this:

0 <= x <=360
cos 20 = cos x

I was able to solve the sin and tan ones but was unable to solve the cos ones.

Our teacher taught us about the 4 quadrant thing.
He says which other quadrant will the value be positive in.
I managed to correctly calculate them for sin and tan (by counting till the angle was in the specific quadrant.

e.g

sin 60.

Sin 60 will be positive in quad S, so we measure till quad S.
180 - 60 = 120
Hence the answer will be sin 120.

I tried doing it for cos but was unable to get the correct answer. Please Help.

 

[mmm4444bot]

There are four angles between 0º and 360º which give rise to a right triangle oriented such that this triangle contains a 20º angle at the origin.

(Double-click my sloppy image to expand.)

[attachment=0:y1jhft0h]QuadCOS.JPG[/attachment:y1jhft0h]

All four right triangles are the same, so the value of h is the same. Do you see that the absolute value of a is the same for each of the four angles marked in blue?

Consider the sign of the ratio a/h for each of these angles.

Does this make it clear which angle between 20º and 360º has the same cosine value as angle 20º?

~ Mark

 

[footytz]

Yes I do. Thank you very much.