The question reads:
The textbook says to take integral of the upper curve minus the lower curve, but how do you determine which is the upper and which is the lower without graphing?
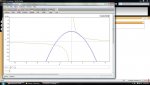
Secondly, so I graphed it, and the graphs are in the attached image. "The area bound by the 2 curves": Wouldn't this be infinity since, in the 3rd quadrant, the area between the 2 curves approaches infinity as y approaches negative infinity?
No intervals nor any other information is given.Find the area bound by the curves
y = -(1/12)x^2 + 7/12 and
y = 1/2x
The textbook says to take integral of the upper curve minus the lower curve, but how do you determine which is the upper and which is the lower without graphing?
Secondly, so I graphed it, and the graphs are in the attached image. "The area bound by the 2 curves": Wouldn't this be infinity since, in the 3rd quadrant, the area between the 2 curves approaches infinity as y approaches negative infinity?