applications to geometry

[thatguy47]

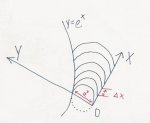
Consider the region bounded by y = ex, the x-axis, the y-axis, and the line x = 3. A solid is created so that the given region is its base and cross-sections perpendicular to the x-axis are semi-circles. Set up a Riemann sum and then a definite integral to find the volume of the solid.


a) What is the volume of a slice perpendicular to the x-axis? (Use Deltax for x as necessary.)

b) What is the exact volume of the solid?

for a) i said (pi)(e^(2x))Deltax which is wrong

for b) i said [integral from 0 to 3] of (pi)(e^(2x))dx which is also wrong

how do i solve this?

 

[mmm4444bot]

for a) i said (pi)(e^(2x))Deltax which is wrong

I think that e^x is the diameter, so e^x/2 is the radius.

Also, don't forget to cut each cylinder in half. In other words, if each slice is a full circle (half on one side of the xy-plane and half on the other side), then the volume of each cylindrical slice is twice what we want because we're only interested in the half of the solid that's on one side of the xy-plane.

I can try to draw a sketch, if you need more help visualizing this object.

 

[thatguy47]

are you saying a would be: (pi)(e^(x/2))DeltaX ??

 

[mmm4444bot]

are you saying a would be: (pi)(e^(x/2))DeltaX ??

No. I'm saying that the radius is e^x/2.

I'm thinking that each slice's volume is half a cylinder:

\(\displaystyle \frac{1}{2} \cdot \pi \cdot \left( \frac{e^x}{2} \right)^2 \cdot \Delta x\)

(I'm scanning a sloppy sketch.)

 

[thatguy47]

thats correct, thanks for your help

 

[mmm4444bot]

?
Double-click image, to expand.

[attachment=0:15b05i96]FunkySolid.JPG[/attachment:15b05i96]

 

[BigGlenntheHeavy]

\(\displaystyle Thanks \ to \ the \ graciousness \ of \ mmm4444bot.'s \ graph, \ we \ have:\)

\(\displaystyle a) \ Area \ (not \ volume) \ of \ a \ slice \ = \ A \ = \ \frac{\pi e^{2x}}{8} \ sq. \ units \ and \ (b), \ the \ volume\)

\(\displaystyle of \ the \ solid \ = \ V \ = \ \frac{\pi}{8}\int_{0}^{3}e^{2x}dx \ = \ \frac{\pi}{16}(e^{6}-1) \ \dot= \ 79.017 \ cu. \ units\)

\(\displaystyle Note: \ A_{semicircle} \ = \ \frac{\pi r^{2}}{2}, \ d \ = \ e^{x}, \ r \ = \ \frac{e^{x}}{2}\)

 

[BigGlenntheHeavy]

\(\displaystyle Post Script:\)

\(\displaystyle V \ = \ \frac{\pi}{8}\int_{0}^{3}e^{2x}dx, \ \Delta x \ = \ \frac{b-a}{n} \ = \ \frac{3-0}{n} \ = \ \frac{3}{n}, \ c_i \ = \ 0+\frac{3i}{n}, \ Right \ Endpoint\)

\(\displaystyle Hence, \ V \ = \ \lim_{||\Delta||\to0}\sum_{i=1}^{n}f(c_i)\Delta x_i \ = \ \lim_{n\to\infty}\frac{\pi}{8}\sum_{i=1}^{n} e^{6i/n}\bigg(\frac{3}{n}\bigg) \ = \ \frac{(e^{6}-1)\pi}{16} \ \dot= \ 79.017\)

\(\displaystyle Here \ we \ show \ both, \ the \ definite \ integral \ and \ its \ Riemann \ sum.\)