Applications of Integration

[tkvictim]

I have a few more problems, and I really need to make sure I know how to do this! My AP calculus class uses relatively simple calculus, I assume, since we didn't even learn Integration by Parts; so any assistance and showing every step will reaaaalllly help. :]

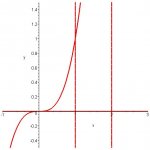
Find the volume of a solid obtained by rotating the region bounded by the curves y=x[sup:1wkht64s]3[/sup:1wkht64s], y=0, and x=1 about the line x=2. Sketch a graph.

I sketched it, I've got something that looks like a small volcano.

 

[BigGlenntheHeavy]

\(\displaystyle 2) \ Disc:\)

\(\displaystyle V \ = \ \pi \int_{0}^{6}[3^{2}-(y/2)^{2}]dy \ = \ 36\pi\)

\(\displaystyle Shells:\)

\(\displaystyle V \ = \ 2\pi \int_{0}^{3} (x)(2x)dx \ = \ 36\pi\)

\(\displaystyle See \ graph:\)

[attachment=0:9fo45254]stu.jpg[/attachment:9fo45254]

 

[tkvictim]

Thank you, I got that

Now I'm just having trouble with that last one. I'm not sure what is the inner and outer radius?

 

[BigGlenntheHeavy]

\(\displaystyle Washer:\)

\(\displaystyle V \ = \ \pi \int_{0}^{1}[(y^{1/3}-2)^{2}-1]dy \ = \ \frac{3\pi}{5}\)

\(\displaystyle Shell:\)

\(\displaystyle V \ = \ 2\pi \int_{0}^{1}(2-x)x^{3}dx \ = \ \frac{3\pi}{5}\)

\(\displaystyle See \ graph:\)

[attachment=0:n6gfnpef]vwx.jpg[/attachment:n6gfnpef]

 

[tkvictim]

Thanks, glenn.

How would you suggest solving this one? Trapezoid rule, midpoint?

The following table shows the velocity of a car (mi/h) during the first five seconds of a race.

t (s) 0 1 2 3 4 5
v (mi/h) 0 20 32 46 54 64

Determine the avg velocity of this car during this five second interval.

I used the midpoint rule, and got 25/2 mi/h.

S .5 (0 + 2[1+2+3+4] +5]
=.5(25)
=25/2

 

[BigGlenntheHeavy]

Hey, pal, what are you, a bottomless pit?

\(\displaystyle Average \ = \ \frac{25}{2} \ = \ 12.5 \ mph, \ I \ don't \ think \ so.\)