application of integration

[evaeswari87]

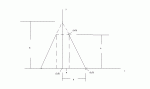
the question is a cylindrical hole of radius a is bored through a solid right-circular cone of height h and base radius b>a.If the axis of the hole lies along that of the cone,find the volume of the remaining part of the cone.

I have tried the question and i got the volume of the large cone as 1/3*pi*b^2*h but i can seem to find the volume of the small cone and cylinder as i can find the height of the cylinder as well as the height of the small cone.can someone pls help with this question.thank you.

my next question is find the centre of mass of the solid plate defined by the region in the xy-plane bounded by y=x^2 and y=x.Assuming that the density of the plate is proportional to the distance from the x-axis and the total mass of the region is M.

for this question,am I suppose to assume the density is a constant or 1??when i assumed it was 1, i got the total mass as 1/6 and the centre of mass is (1/2,2/5).Is my answer right??pls help me double check my answer.thank you :?:

 

[galactus]

I will let the height of the drilled cylindrical portion be h. Then we have a lne formed by the slant of the cone with coordinates (b,0) and (a,h). You can find the equation of this line and use washers to integrate wrt x.

If we set up an integral we get:

\(\displaystyle {\pi}\int_{a}^{b}\left[\frac{h}{a-b}x-\frac{bh}{a-b}\right]^{2}dx\)

Which can be simplified to: \(\displaystyle \frac{h^{2}{\pi}}{(a-b)^{2}}\int_{a}^{b}(x-b)^{2}dx\)

I hope I set that up correctly. Check and see what you get.