Another Trig Question

M

mathfun

Junior Member
Joined
Dec 26, 2005
Messages
89
if sinx + cosx = 1/5 and o<_x<x, then tanx is ?

what i have done is:
cosx = 1/5 -sinx
(cosx)^2 = (1/5 - sinx)^2 = 1 - (sinx)^2

(1/5 - sinx)^2 = 1 - (sinx)^2
25(sinx)^2 - 5sinx -12 = 0
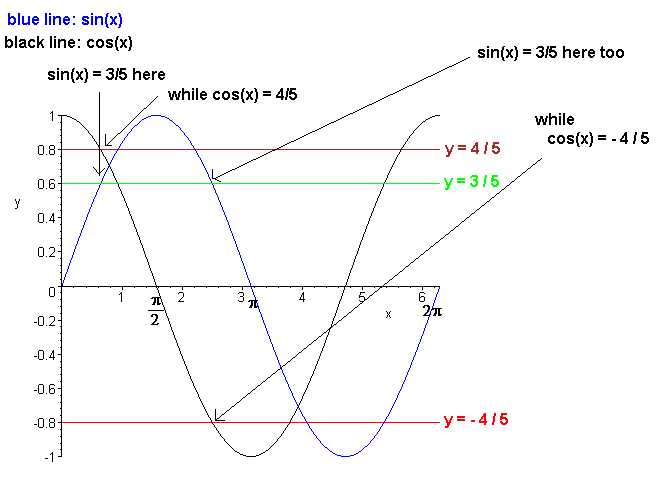
sinx= 4/5, -3/5 <--rejected
sinx = 4/5

put it back into the equation: (cosx)^2 = (1/5 - sinx)^2 = 1 - (sinx)^2
(cosx)^2 = (1/5 - 4/5)^2 = 1 - (4/5)^2
(cosx)^2 = 1 - (4/5)^2
=1- 16/25
=9/25
=squareroot(9/25)
cosx=3/5

draw the right triangle and tan it.
tanx=4/3

that is the answer i got, however, the answer is -4/3. :?
 
If sin(x) = 4/5 and sin(x) + cos(x) = 1/5, what is the sign of cos(x)?

You are not in Quadrant I.

Similarly, if ccos(x) = 4/5 and sin(x) + cos(x) = 1/5, what is the sign of sin(x)?

You're still not in Quadrant I

Your triangle will give you the reference angle. It will not tell you what Quadrant to put it in.
 
since i already found sinx, and i need tanx, why can't i just go straight from there? Why do i have to instead plug it back in to the equation (sinx+cosx=1/5) and find cosx and then tanx?
 
That's the problem with periodic functions. You can't always tell where you are. Figuring out where you are is well more than half the fun.

There are infinitely many solutions in many cases. Picking out exactly which one(s) you want is the important part.
 
instead of starting another topic, i decided just to add to this one since its trig anyways.

((cos(x/2))^2 + 2cos(x/2)sin(x/2) + (sin(x/2))^2)) -1
= 2cos(x/2)sin(x/2)

how does that work?
 
You should know these:

(x+y)^2 = x^2 + 2*x*y + y^2

[sin(x)]^2 + [cos(x)]^2 = 1

They will get you through just about anything you could possibly want to know about this one.
 
yea...eggnog is yummy. :D

anyway, since for the first question... i already found sinx, and i need tanx, i had to plug it back in to the equation (sinx+cosx=1/5) and find cosx and then tanx.

then...for example..
if sinx=(3/5) and i want to find cosx... how do i do that?

i started doing it like this: (i'm not sure if i can do this...)
(sinx)^2 + (cosx)^2 = 1
(3/5)^2 - 1 =- (cosx)^2
therefore.. cosx should = 4/5

however, my teacher said its -4/5.....where did the negative come from?

(btw, this is part of a whole long longlong long difficult process of a question)
 
It depends on the domain.

sin(x) is positive in quadrants 1 and 2, so the domain could be 0<x<pi/2 or pi/2<x<pi.

cos(x) is positive in the first of those domains and negative in the latter.
 
A plot may help clarify things.

In the domain 0 to 2pi:

mathfun2.gif
 
You must log in or register to reply here.
Top