MathsFormula
Junior Member
- Joined
- Jul 13, 2014
- Messages
- 95
A large circle has a radius of 10 cm. Contained within this circle are four smaller circles of equal size (fitting inside the larger circle exactly). The question asks: Find the radius of the largest circle which will fit in the middle.
Here is a link to a picture that I’ve pulled from Google that shows the same diagram as the maths book:
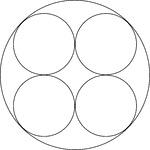
The book answer is: 1.72 cm
My attempt: The distance between the points of touch of the smaller circles is: 20/3 = 6.66 cm
So the distance between the point of touch of one of the smaller circles and the centre of the larger circle is: 6.66/2 = 3.33 cm
Therefore the radius of the smaller circle should also be 3.33 cm
Total diameter of two circles facing diagonally opposite each other is 3.33 x 4 = 13.32 cm
So the distance remaining between the circles is 20 – 13.32 = 6.68 cm
So radius if a circle in the middle would be 6.68/2 = 3.34 cm THIS IS THE WRONG ANSWER
Here is a link to a picture that I’ve pulled from Google that shows the same diagram as the maths book:

The book answer is: 1.72 cm
My attempt: The distance between the points of touch of the smaller circles is: 20/3 = 6.66 cm
So the distance between the point of touch of one of the smaller circles and the centre of the larger circle is: 6.66/2 = 3.33 cm
Therefore the radius of the smaller circle should also be 3.33 cm
Total diameter of two circles facing diagonally opposite each other is 3.33 x 4 = 13.32 cm
So the distance remaining between the circles is 20 – 13.32 = 6.68 cm
So radius if a circle in the middle would be 6.68/2 = 3.34 cm THIS IS THE WRONG ANSWER
Last edited by a moderator:
