Adding and Subtracting Rational Functions

What is a rational function again?

As a reminder, a rational function is an expression formed by dividing two polynomial functions. For example, the following is a rational function:

$f(x)=\frac{4x+4}{6x-9}$

How do we add or subtract them?

When adding or subtracting rational functions, you must find a common denominator as you might do with regular fractions. For example, to add $\frac{1}{2}$ and $\frac{1}{3}$ , you might do the following:

The original fractions: $\frac{1}{2}+\frac{1}{3}$

with a common denominator: $\frac{2}{6}+\frac{3}{6}$

result: $\frac{5}{6}$

Now, let's apply this same strategy to the addition and subtraction of rational functions:

$\frac{x+1}{x+3}+\frac{2x+5}{x-2} = ??$

Step 1

Find a common denominator by multiplying the denominators. So, $(x + 3)(x - 2)$ becomes our common denominator in this case. Then, multiply each fraction by something equivalent to "1" (which of course doesn't change the actual value!), such as $\frac{x+3}{x+3}$ , to get each fraction in terms of that common denominator:

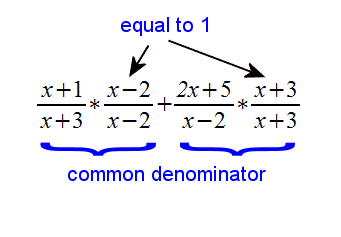
Rational Functions

Step 2

Now, multiply out (expand) the top and we will be ready to add the two fractions:

$\frac{(x+1)(x-2)}{(x+3)(x-2)}+\frac{(2x+5)(x+3)}{(x+3)(x-2)}$

$\frac{x^2-2x+x-2}{(x+3)(x-2)}+\frac{2x^2+6x+5x+15}{(x+3)(x-2)}$

Step 3

Add the numerators just like you would with two simple fractions:

$\frac{3x^2+10x+13}{(x+3)(x-2)}$

The Result

Finally, we probably want to expand the denominator as well to give us the resulting rational function:

$\frac{3x^2+10x+13}{x^2+x-6}$

And that's our answer!

NOTE: To subtract rational functions, follow the same steps that you used to add rational functions, but just subtract the numerators instead of adding them!

For information on rational functions, try a search on Google or this lesson on rational functions.

By Mr. Feliz and Free Math Help